+ - 0:00:00
Notes for current slide
Notes for next slide

1.4 — Preferences and Utility

ECON 306 • Microeconomic Analysis • Fall 2020

Ryan Safner
Assistant Professor of Economics
safner@hood.edu
ryansafner/microF20
microF20.classes.ryansafner.com

Outline

Preferences

Indifference Curves

Marginal Rate of Substitution

Utility

Marginal Utility

MRS and Preferences

Consumer's Objectives

  • What do consumers want? What do they maximize?

  • Avoid being normative & make as few assumptions as possible

  • We'll assume people maximize preferences

    • WTF does that mean?

Preferences

Preferences I

  • Which bundles of (x,y) are preferred over others?

Example:

a=(412) or b=(612)

Preferences II

  • We will allow three possible answers:

Preferences II

  • We will allow three possible answers:
  1. a≻b: Strictly prefer a over b

Preferences II

  • We will allow three possible answers:

  1. a≻b: Strictly prefer a over b

  2. a≺b: Strictly prefer b over a

Preferences II

  • We will allow three possible answers:

  1. a≻b: Strictly prefer a over b

  2. a≺b: Strictly prefer b over a

  3. a∼b: Indifferent between a and b

Preferences II

  • We will allow three possible answers:

  1. a≻b: Strictly prefer a over b

  2. a≺b: Strictly prefer b over a

  3. a∼b: Indifferent between a and b

  • Preferences are a list of all such comparisons between all bundles

See appendix in today's class page for more.

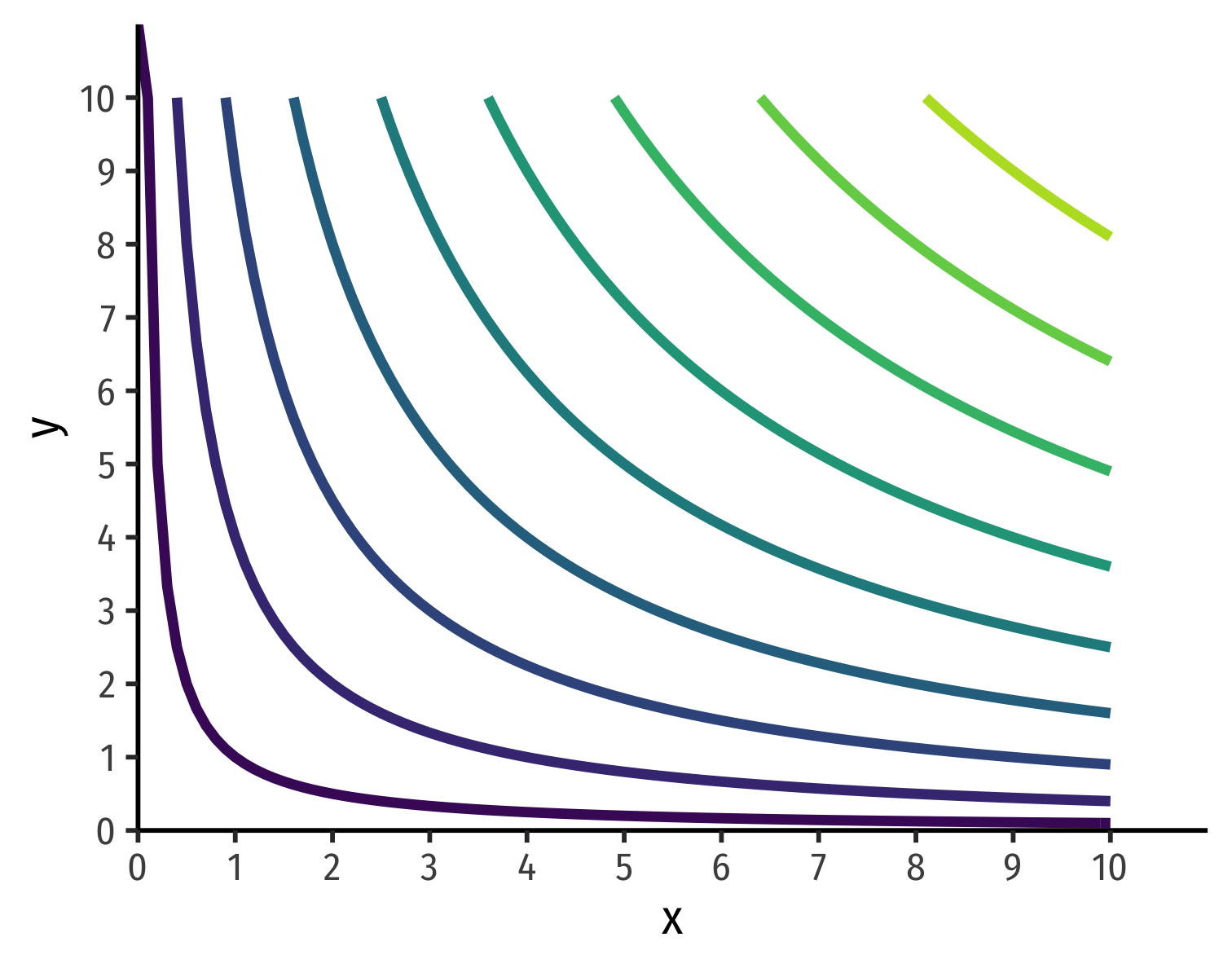
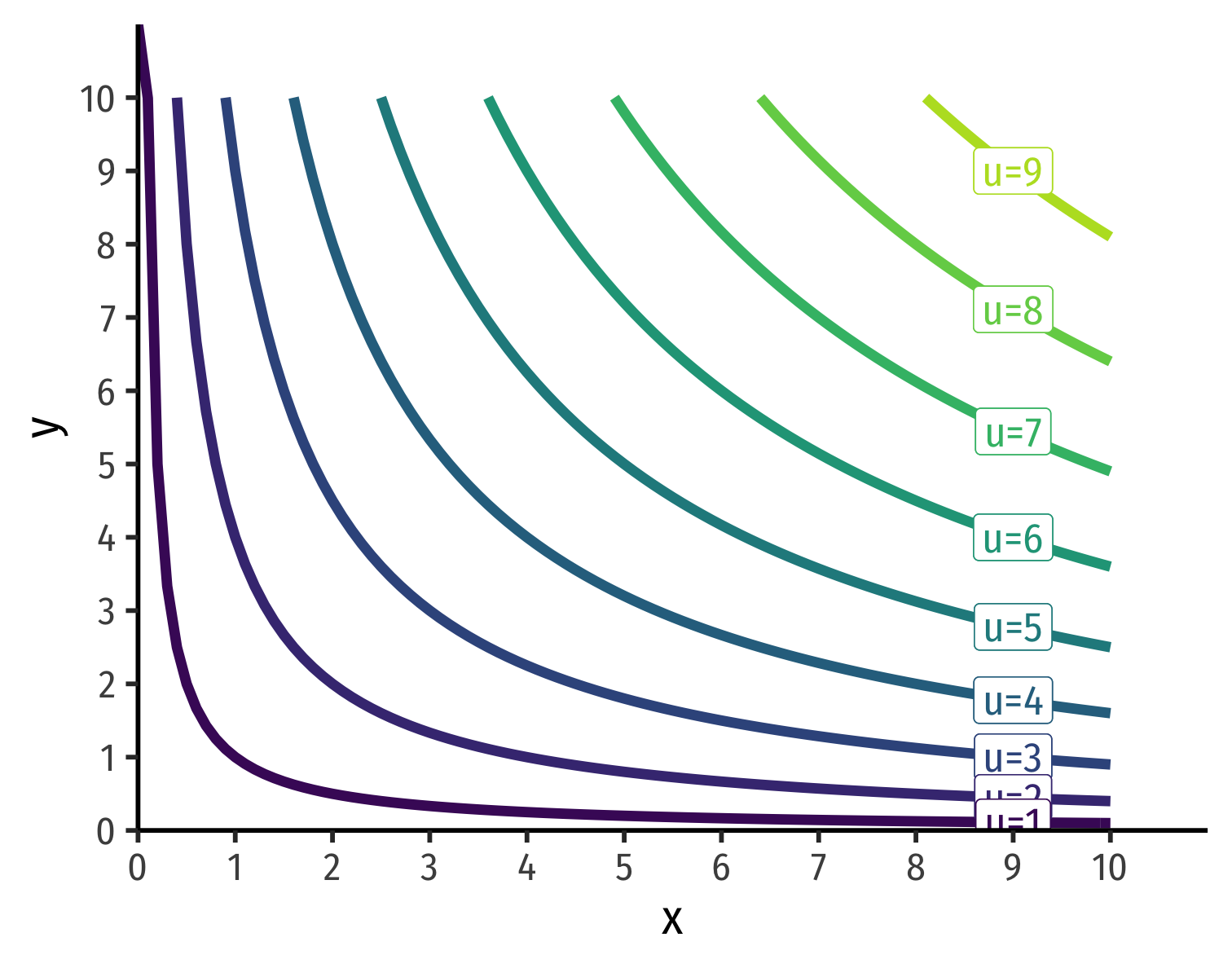
Indifference Curves

Mapping Preferences Graphically I

  • For each bundle, we now have 3 pieces of information:

    • amount of x
    • amount of y
    • preference compared to other bundles

  • How to represent this information graphically?

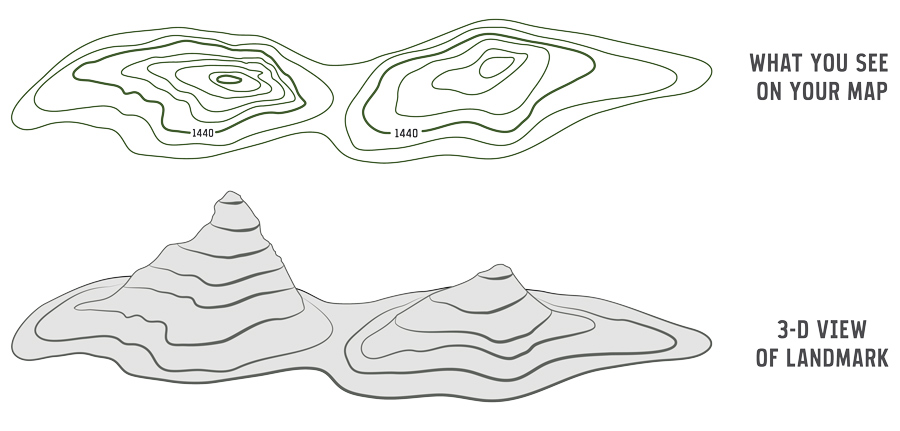
Mapping Preferences Graphically II

  • Cartographers have the answer for us

  • On a map, contour lines link areas of equal height

  • We will use "indifference curves" to link bundles of equal preference

Mapping Preferences Graphically III

3-D "Mount Utility"

2-D Indifference Curve Contours

Indifference Curves: Example

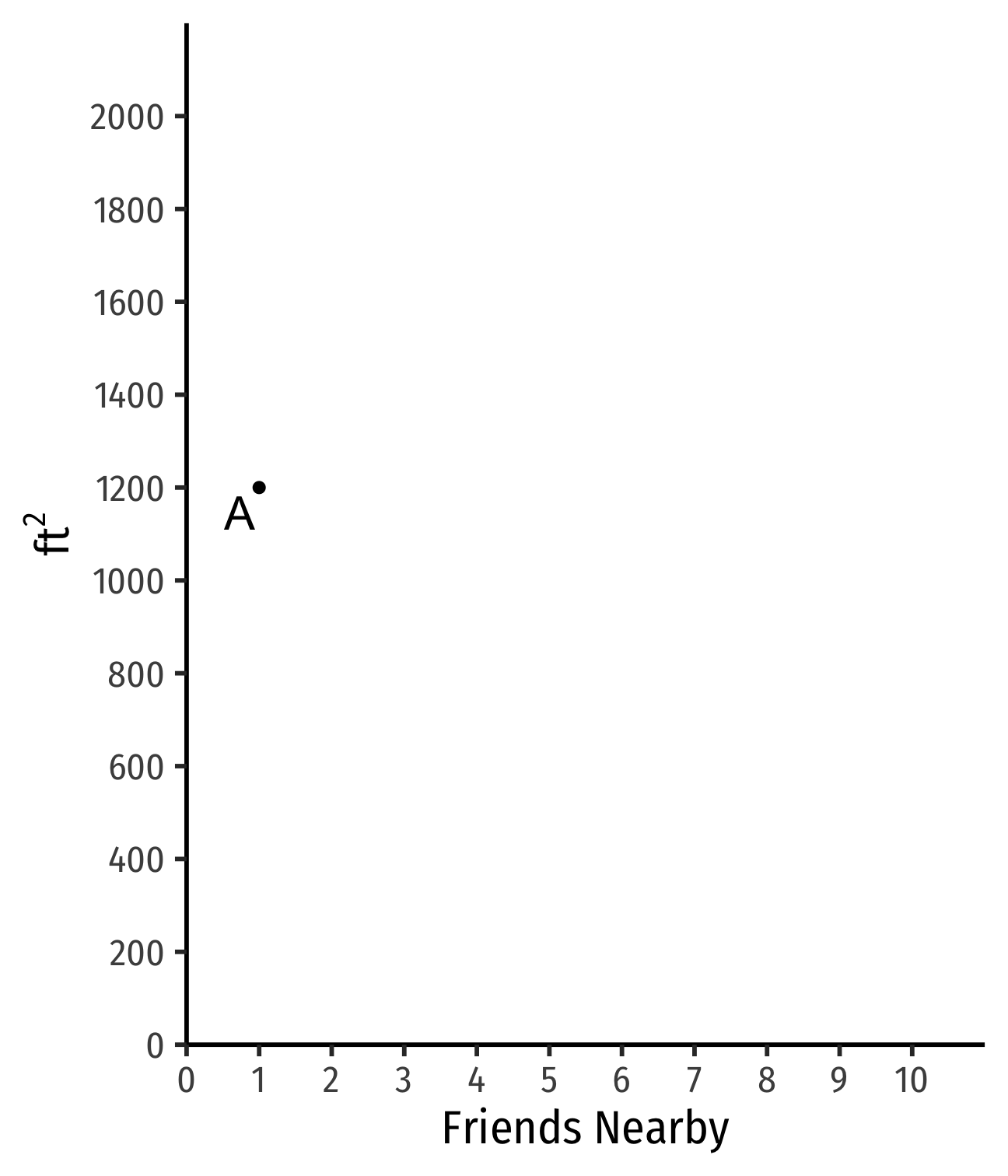
Example: Suppose you are hunting for an apartment. You value both the size of the apartment and the number of friends that live nearby.

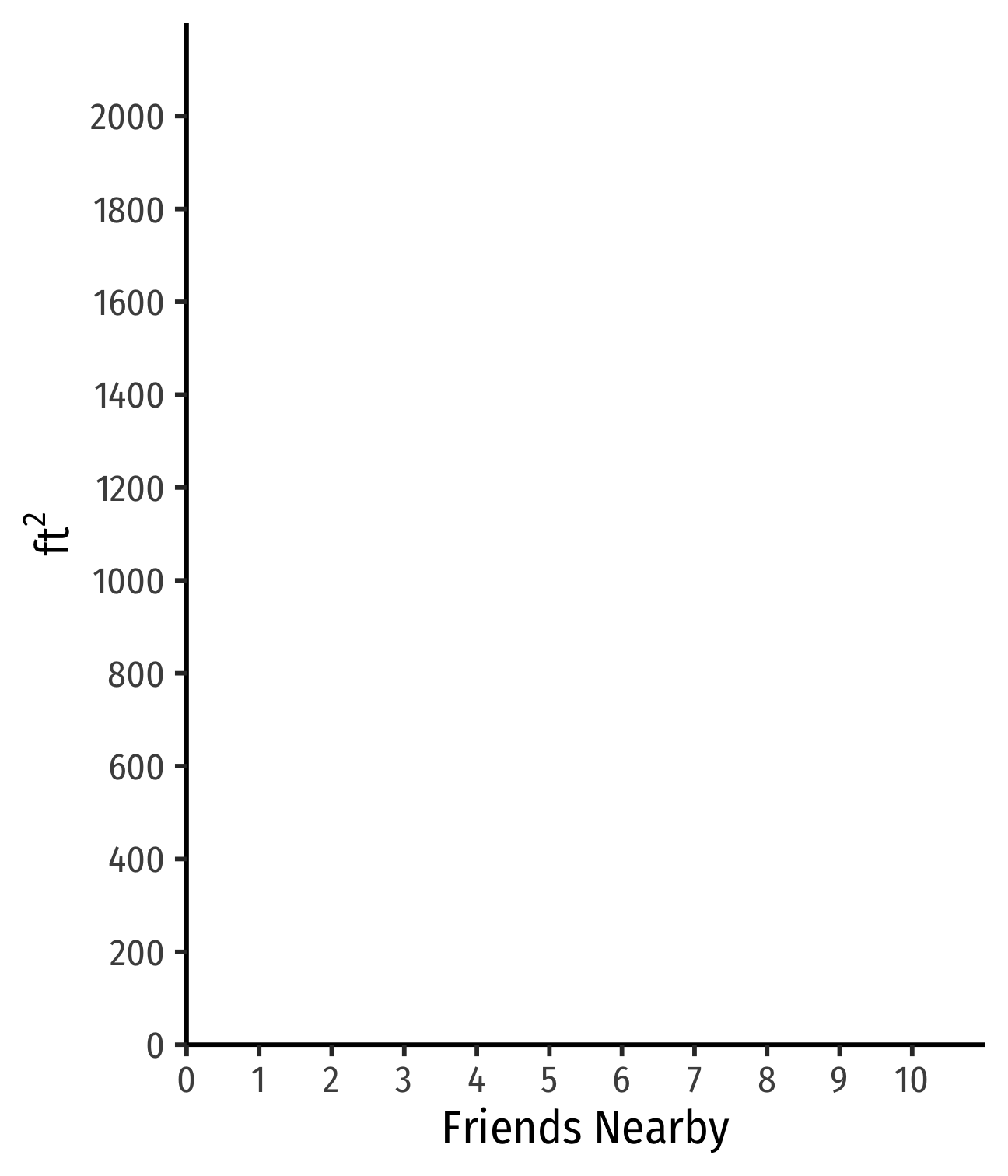
Indifference Curves: Example

Example: Suppose you are hunting for an apartment. You value both the size of the apartment and the number of friends that live nearby.

  • Apt. A has 1 friend nearby and is 1,200 ft2

Indifference Curves: Example

Example: Suppose you are hunting for an apartment. You value both the size of the apartment and the number of friends that live nearby.

  • Apt. A has 1 friend nearby and is 1,200 ft2
    • Apartments that are larger and/or have more friends ≻A

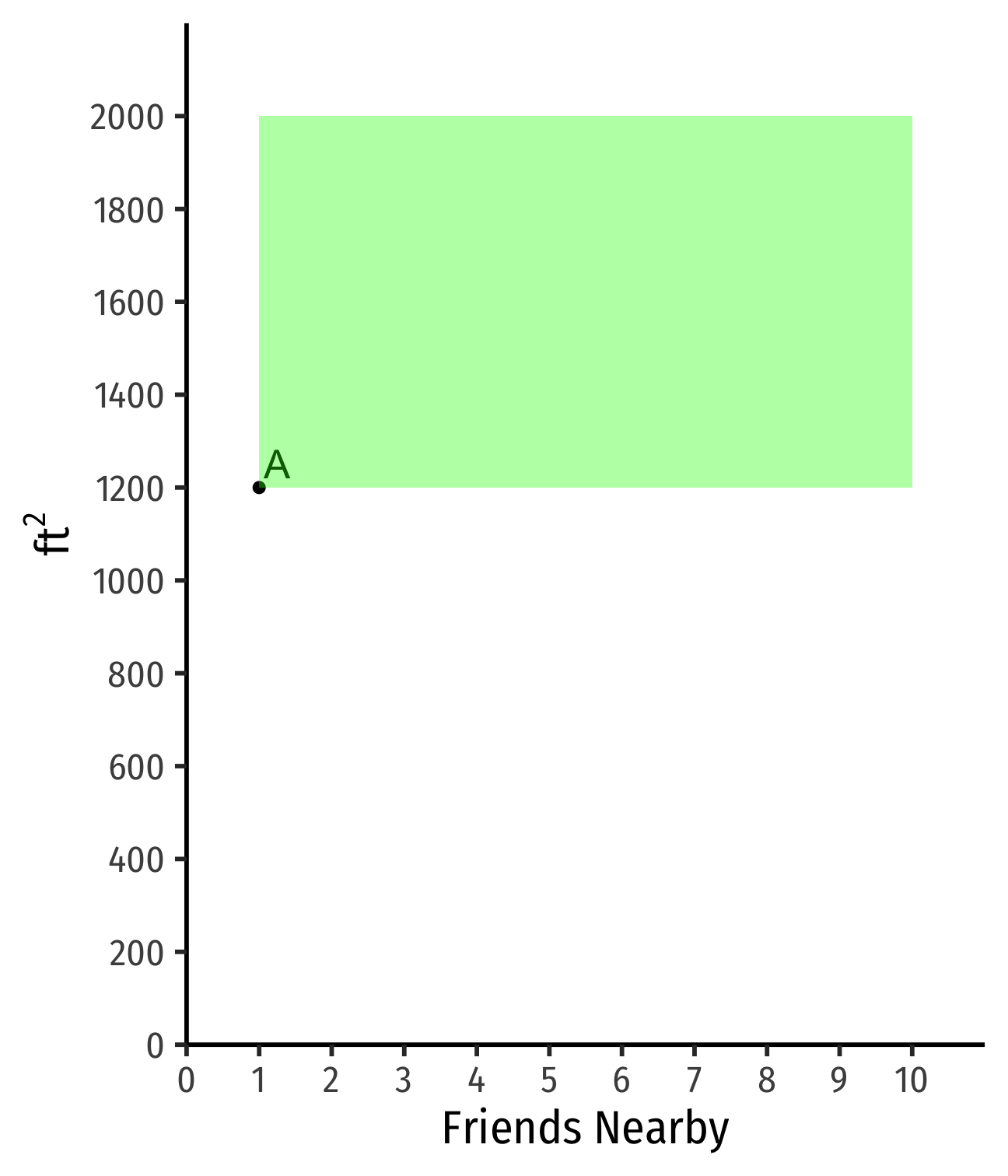
Indifference Curves: Example

Example: Suppose you are hunting for an apartment. You value both the size of the apartment and the number of friends that live nearby.

  • Apt. A has 1 friend nearby and is 1,200 ft2
    • Apartments that are larger and/or have more friends ≻A
    • Apartments that are smaller and/or have fewer friends ≺A

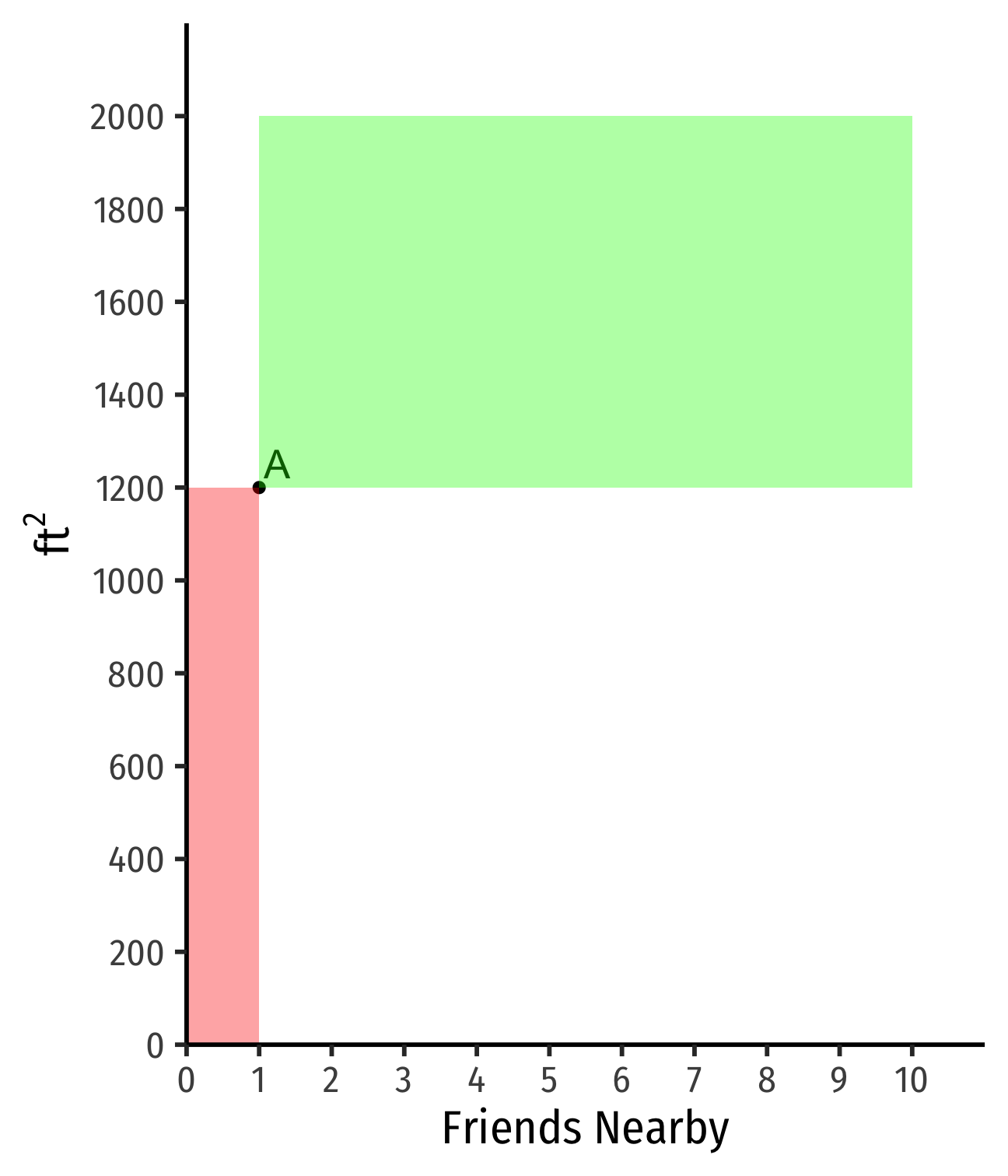
Indifference Curves: Example

Example:

  • Apt. A has 1 friend nearby and is 1,200 ft2

  • Apt. B has more friends but less ft2

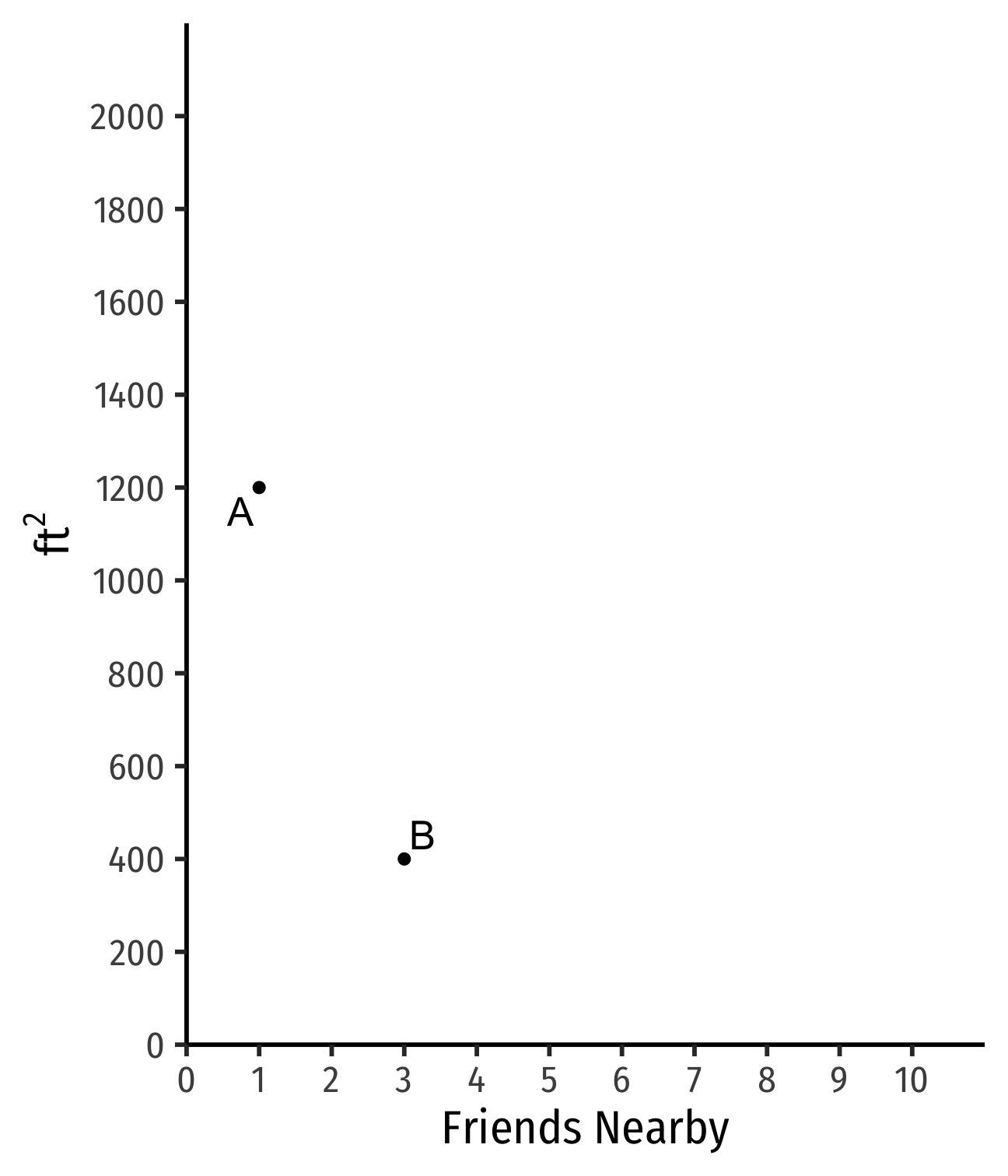
Indifference Curves: Example

Example:

  • Apt. A has 1 friend nearby and is 1,200 ft2

  • Apt. B has more friends but less ft2

  • Apt. C has still more friends but less ft2

Indifference Curves: Example

Example:

  • Apt. A has 1 friend nearby and is 1,200 ft2

  • Apt. B has more friends but less ft2

  • Apt. C has still more friends but less ft2

  • If A∼B∼C, on same indifference curve

Indifference Curves: Example

  • Indifferent between all apartments on the same curve

Indifference Curves: Example

  • Indifferent between all apartments on the same curve

  • Apts above curve are preferred over apts on curve

    • D≻A∼B∼C
    • On a higher curve

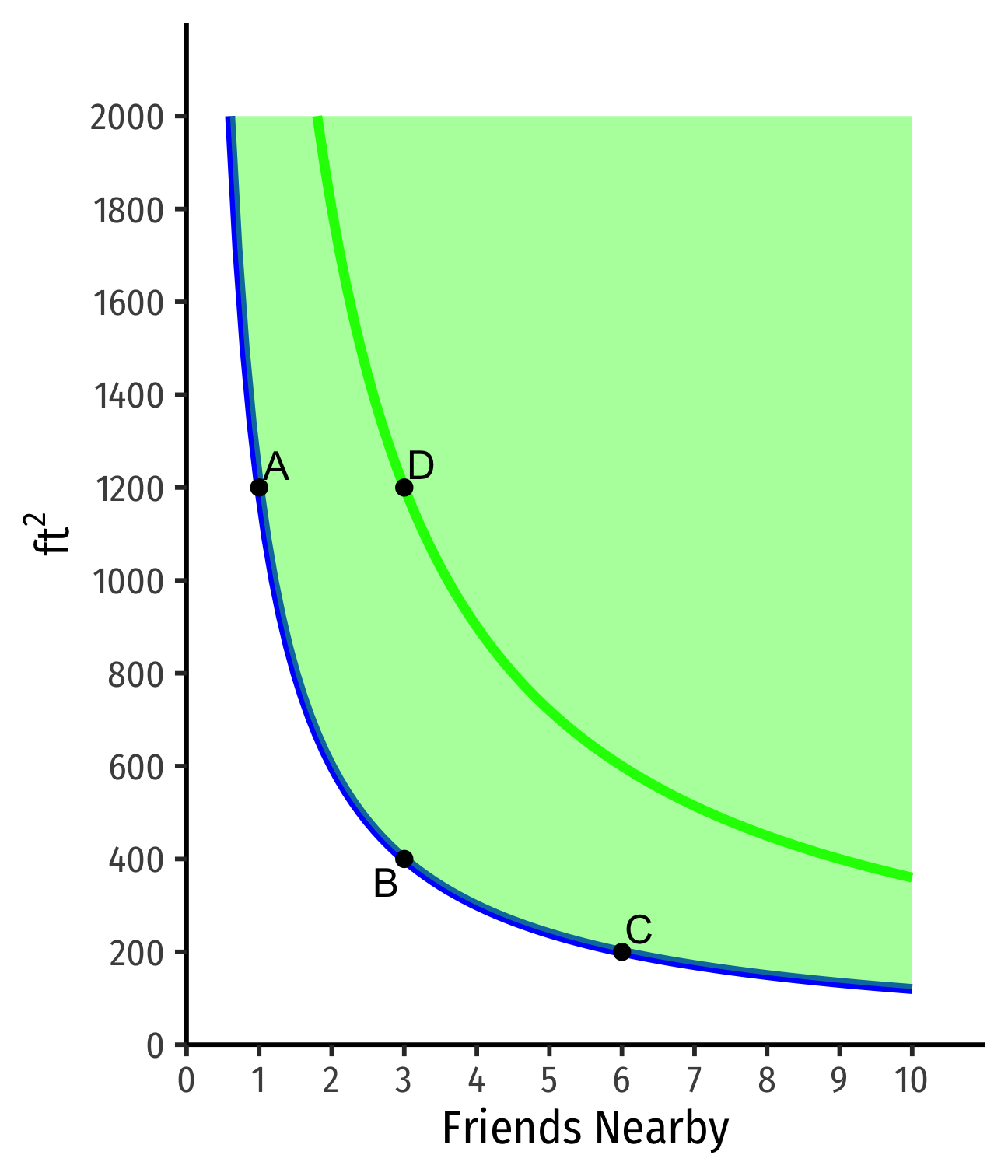
Indifference Curves: Example

  • Indifferent between all apartments on the same curve

  • Apts above curve are preferred over apts on curve

    • D≻A∼B∼C
    • On a higher curve
  • Apts below curve are less preferred than apts on curve
    • E≺A∼B∼C
    • On a lower curve

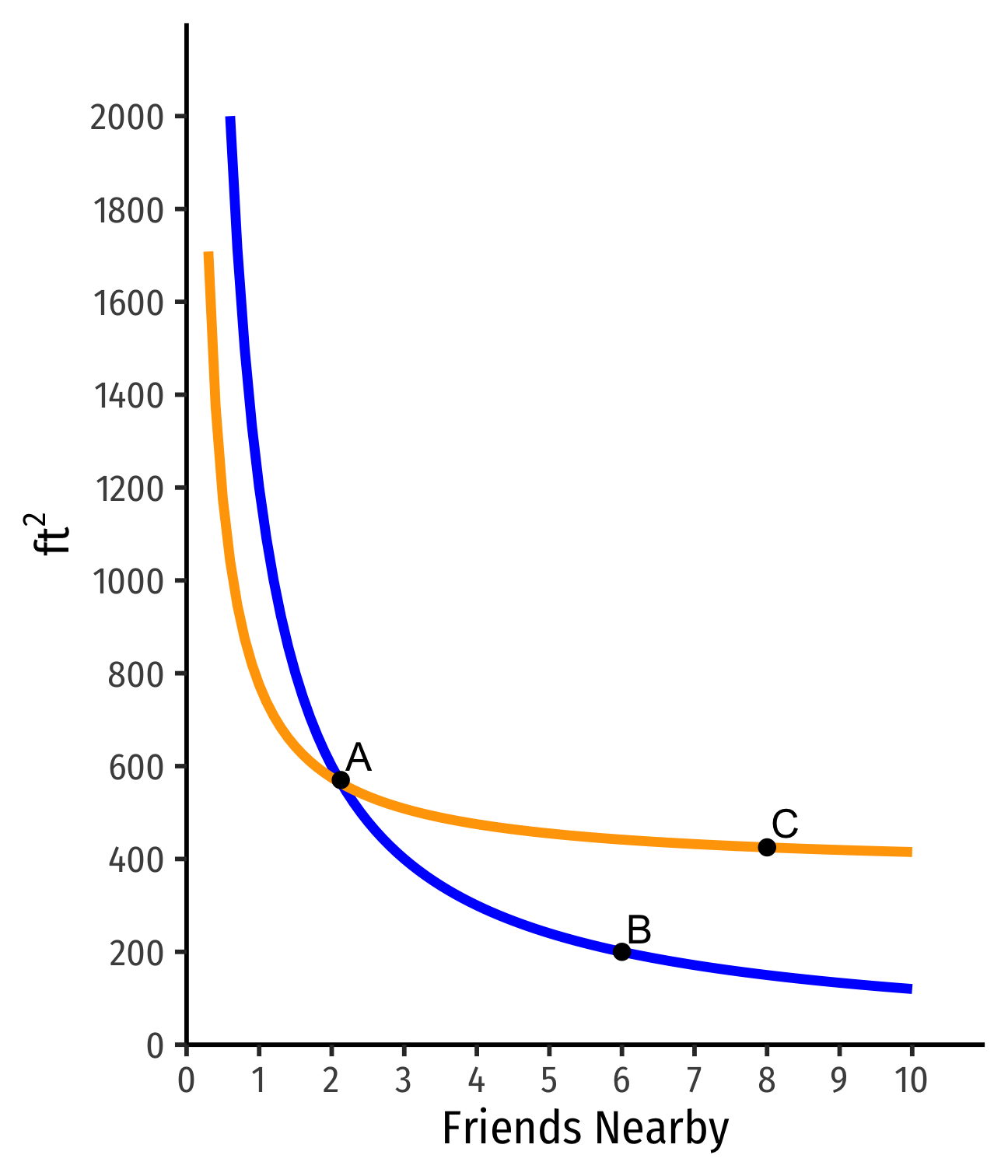
Curves Never Cross!

  • Indifference curves can never cross: preferences are transitive
    • If I prefer A≻B, and B≻C, I must prefer A≻C

Curves Never Cross!

  • Indifference curves can never cross: preferences are transitive

    • If I prefer A≻B, and B≻C, I must prefer A≻C

  • Suppose two curves crossed:

    • A∼B
    • B∼C
    • But C ≻ B!
    • Preferences are not transitive!

Marginal Rate of Substitution

Marginal Rate of Substitution I

  • If I take away one friend nearby, how many more ft2 would you need to keep you indifferent?

Marginal Rate of Substitution I

  • If I take away one friend nearby, how many more ft2 would you need to keep you indifferent?

  • Marginal Rate of Substitution (MRS): rate at which you trade off one good for the other and remain indifferent

  • Think of this as your opportunity cost: # of units of y you need to give up to acquire 1 more x

MRS vs. Budget Constraint Slope

  • Budget constraint (slope) measured the market's tradeoff between x and y based on market prices

  • MRS measures your personal evaluation of x vs. y based on your preferences

  • Foreshadowing: what if they are different? Are you truly maximizing your preferences?

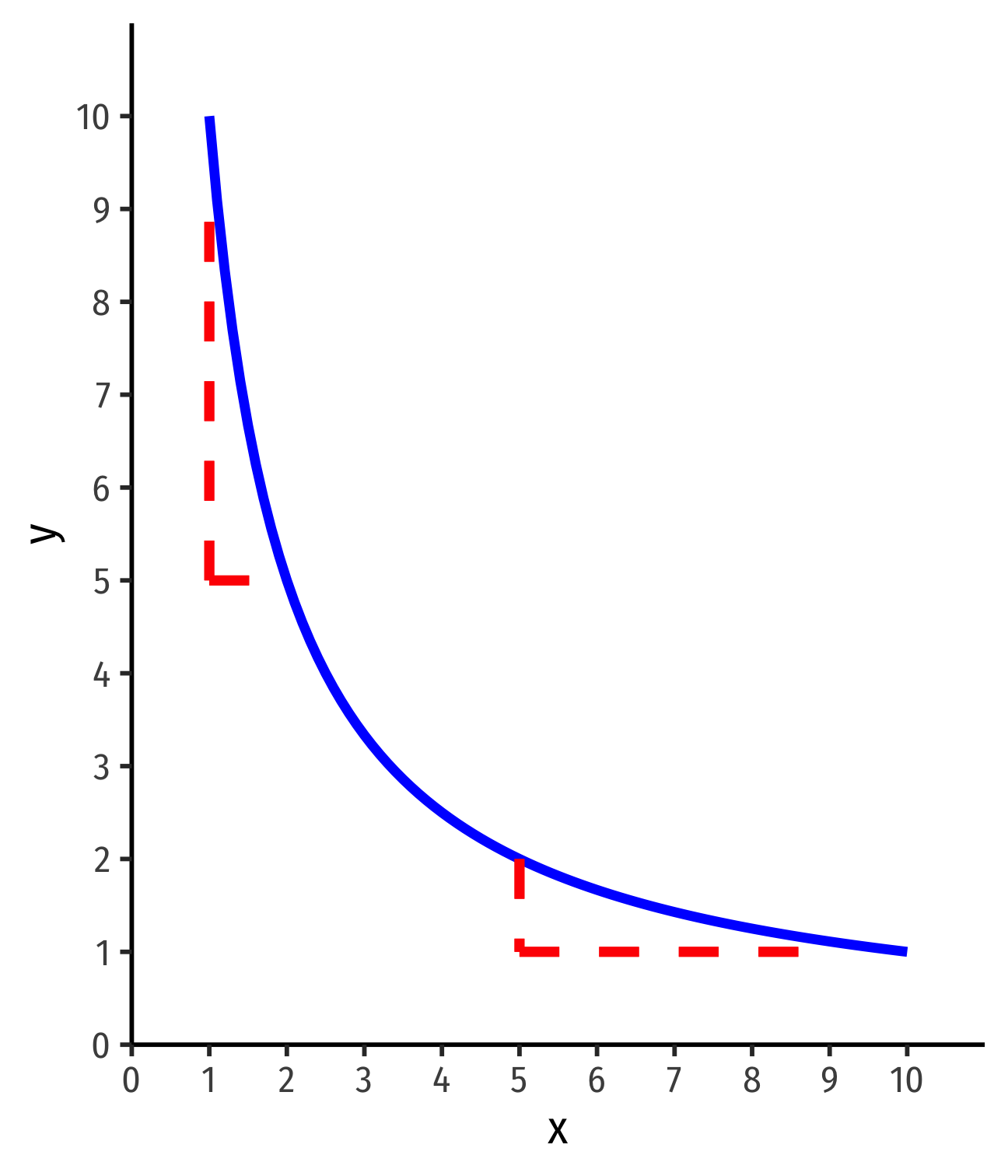
Marginal Rate of Substitution II

  • MRS is the slope of the indifference curve MRSx,y=−ΔyΔx=riserun

  • Amount of y given up for 1 more x

  • Note: slope (MRS) changes along the curve!

Utility

So Where are the Numbers?

  • Long ago (1890s), utility considered a real, measurable, cardinal scale†

  • Utility thought to be lurking in people's brains

    • Could be understood from first principles: calories, water, warmth, etc

  • Obvious problems

† "Neuroeconomics" & cognitive scientists are re-attempting a scientific approach to measure utility

Utility Functions?

  • 20th century innovation: preferences as the objects of maximization

  • We can plausibly measure preferences via implications of peoples' actions!

  • Principle of Revealed Preference: if x and y are both feasible, and if x is chosen over y, then the person must (weakly) prefer x⪰y

  • Flawless? Of course not. But extremely useful!

Utility Functions! I

  • So how can we build a function to "maximize preferences"?

  • Construct a utility function u(⋅)† that represents preference relations (≻,≺,∼)

  • Assign utility numbers to bundles, such that, for any bundles a and b: a≻b⟺u(a)>u(b)

† The ⋅ is a placeholder for whatever goods we are considering (e.g. x, y, burritos, lattes, etc)

Utility Functions! II

  • We can model "as if" the consumer is maximizing utility/preferences by maximizing the utility function:

  • "Maximizing preferences": choosing a such that a≻b for all available b

  • "Maximizing utility": choosing a such that u(a)>u(b) for all available b

  • Identical if they contain the same information

Utility Functions, Pural I

  • Imagine three alternative bundles of (x,y): a=(1,2)b=(2,2)c=(4,3)

Utility Functions, Pural I

  • Imagine three alternative bundles of (x,y): a=(1,2)b=(2,2)c=(4,3)
  • Create a utility function u(⋅) that assigns each bundle a utility level of
u(⋅)
u(a)=1
u(b)=2
u(c)=3

Utility Functions, Pural I

  • Imagine three alternative bundles of (x,y): a=(1,2)b=(2,2)c=(4,3)
  • Create a utility function u(⋅) that assigns each bundle a utility level of
u(⋅)
u(a)=1
u(b)=2
u(c)=3
  • Does it mean that bundle c is 3 times the utility of a?

Utility Functions, Pural II

  • Imagine three alternative bundles of (x,y): a=(1,2)b=(2,2)c=(4,3)
  • Now consider u(⋅) and a second utility function v(⋅):
u(⋅) v(⋅)
u(a)=1 v(a)=3
u(b)=2 v(b)=5
u(c)=3 v(c)=7

Utility Functions, Pural III

  • Utility numbers have an ordinal meaning only, not cardinal

    • Only the ordering c≻b≻a matters!

  • Both are valid:†

    • u(c)>u(b)>u(a)
    • v(c)>v(b)>v(a)

† See the Mathematical Appendix in Today's Class Page for why.

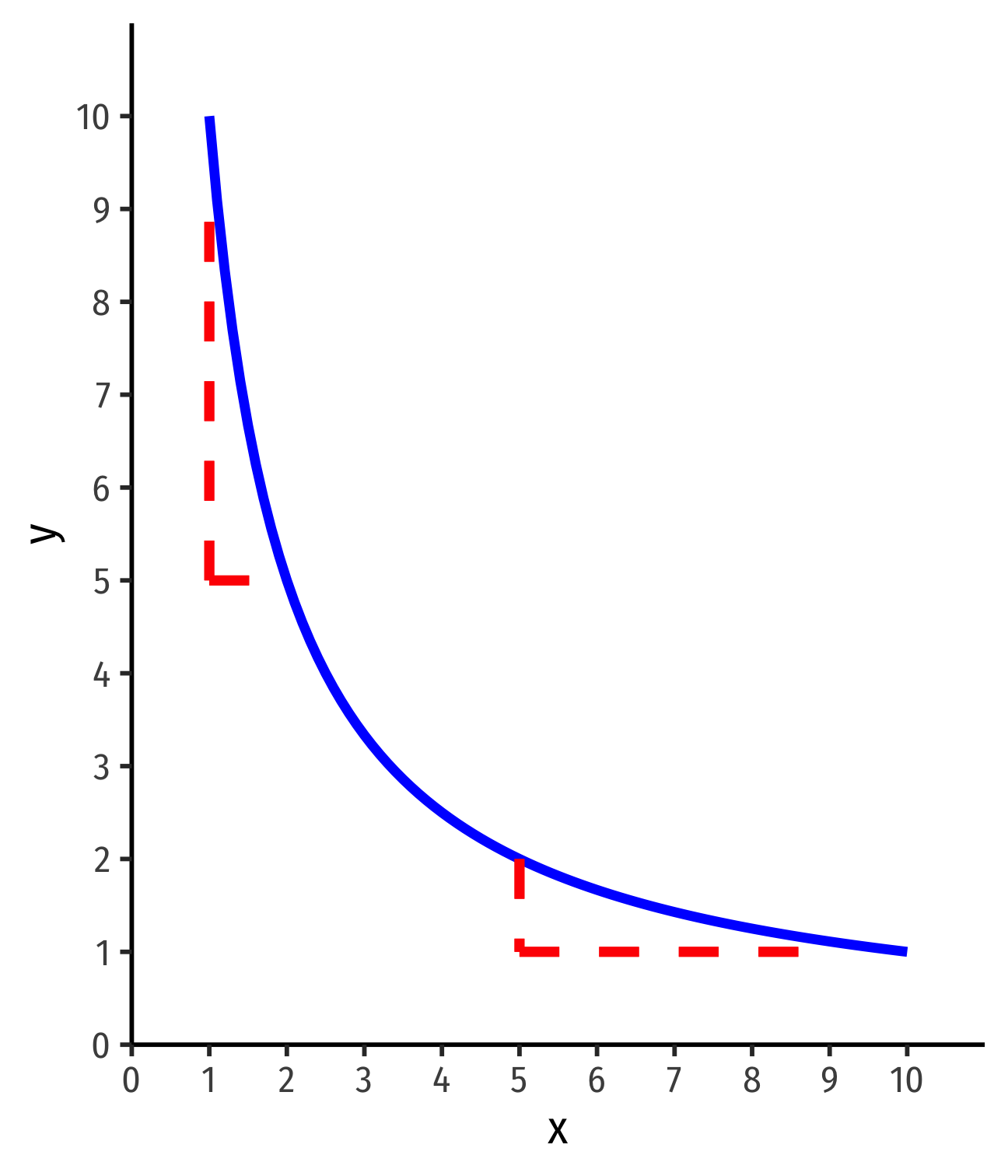
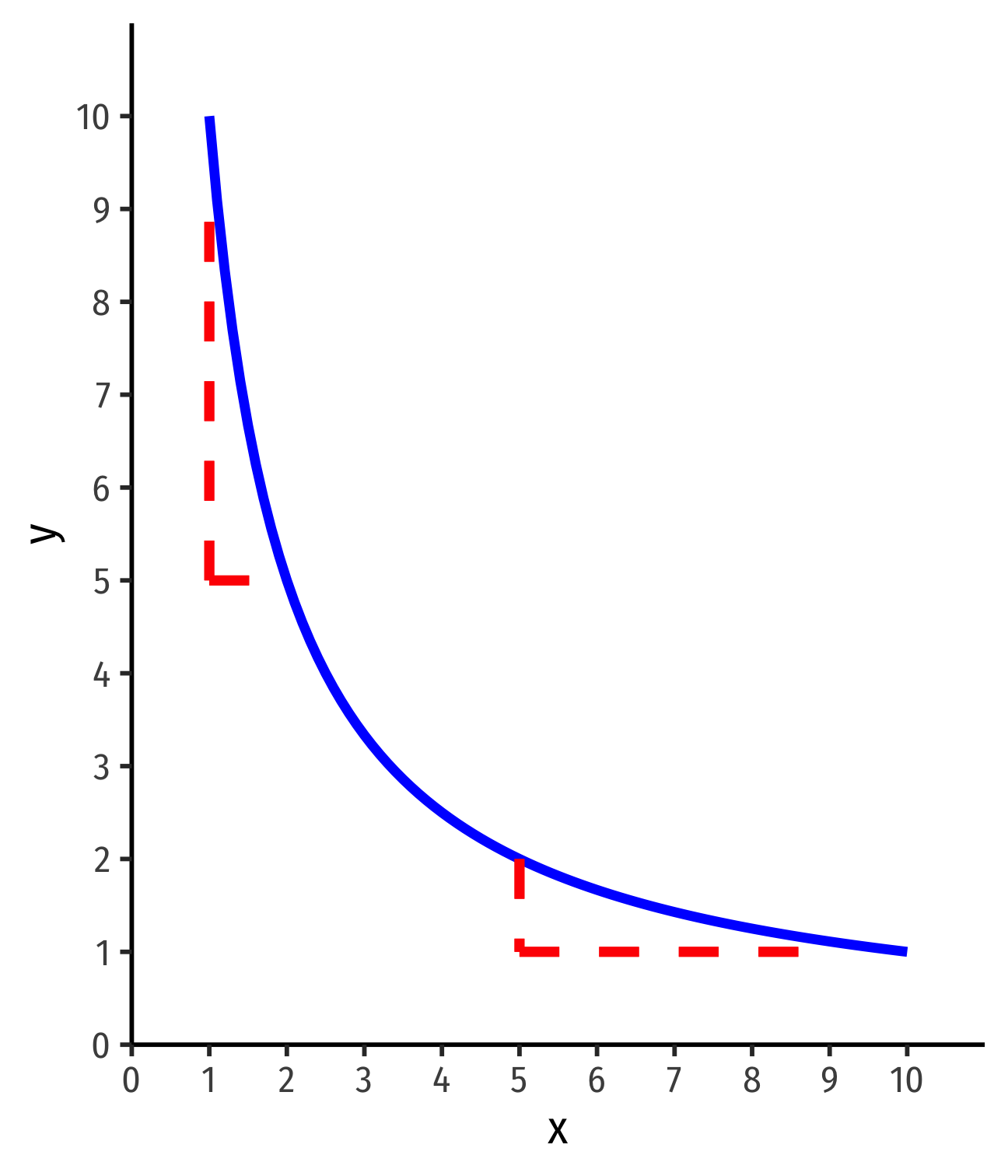
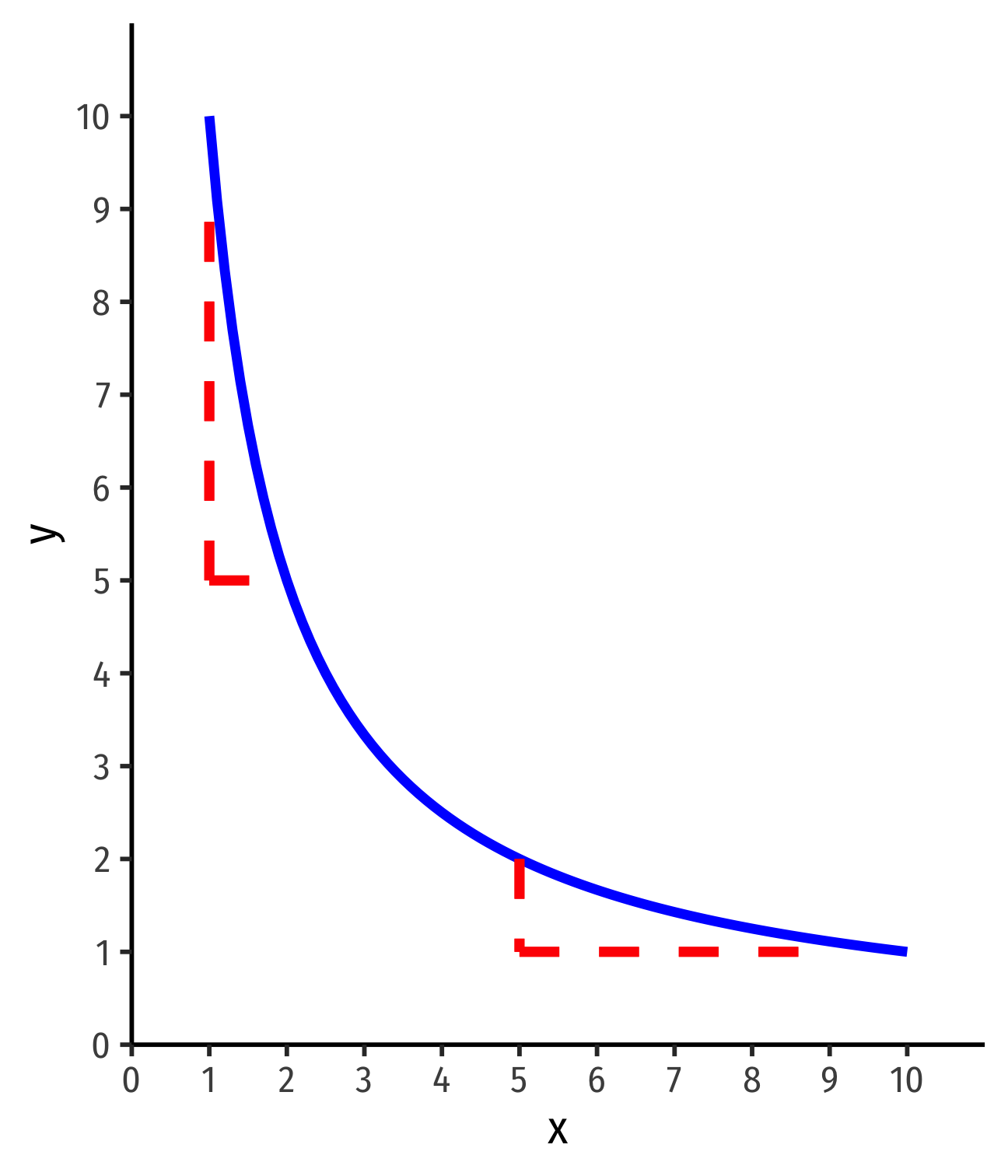
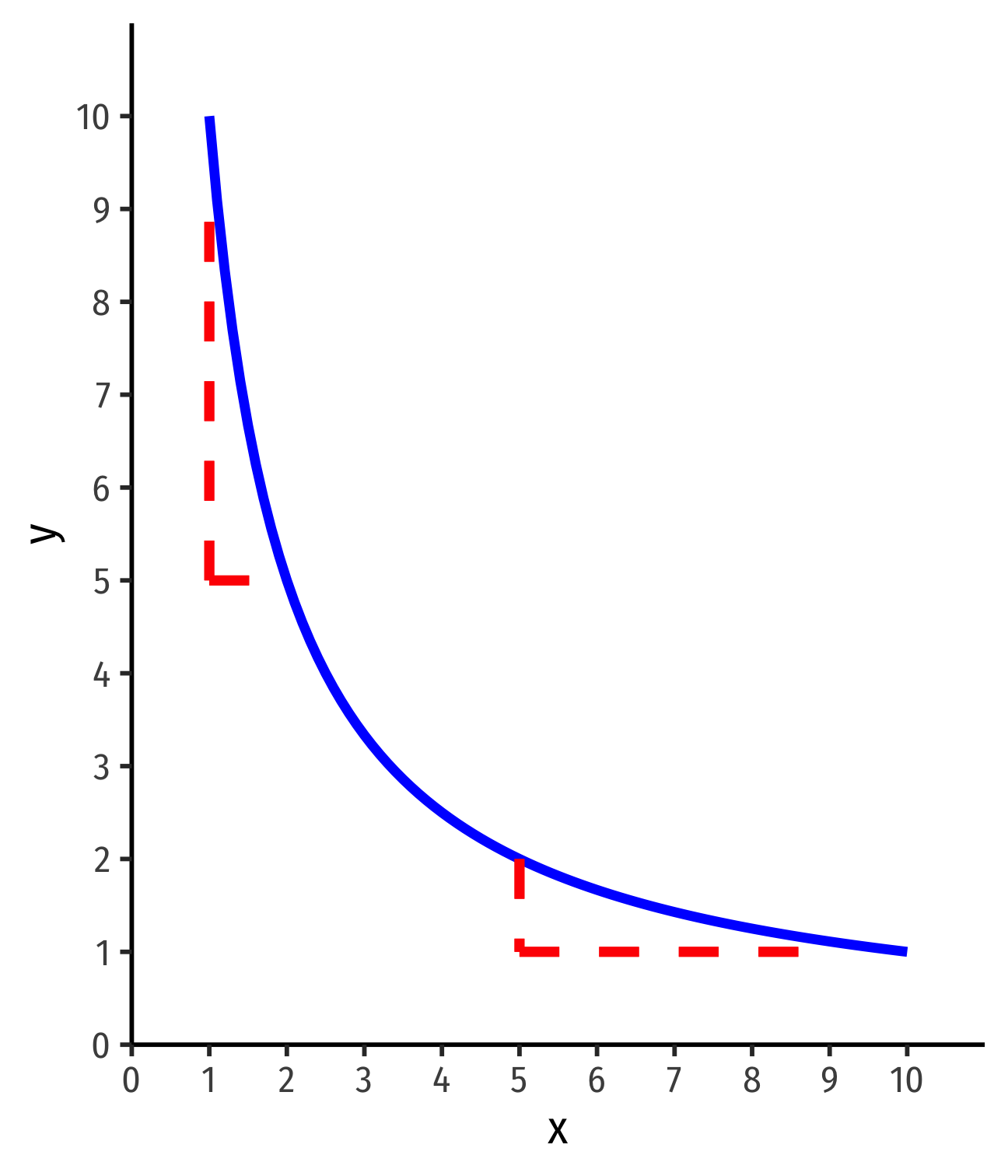
Utility Functions and Indifference Curves I

  • Two tools to represent preferences: indifference curves and utility functions

  • Indifference curve: all equally preferred bundles ⟺ same utility level

  • Each indifference curve represents one level (or contour) of utility surface (function)

Utility Functions and Indifference Curves II

3-D Utility Function: u(x,y)=√xy

2-D Indifference Curve Contours: y=u2x

Marginal Utility

MRS and Marginal Utility I

  • Recall: marginal rate of substitution MRSx,y is slope of the indifference curve

    • Amount of y given up for 1 more x

  • How to calculate MRS?

    • Recall it changes (not a straight line)!
    • We can calculate it using something from the utility function

MRS and Marginal Utility II

  • Marginal utility: change in utility from a marginal increase in consumption

MRS and Marginal Utility II

  • Marginal utility: change in utility from a marginal increase in consumption

Marginal utility of x: MUx=Δu(x,y)Δx

MRS and Marginal Utility II

  • Marginal utility: change in utility from a marginal increase in consumption

Marginal utility of x: MUx=Δu(x,y)Δx

Marginal utility of y: MUy=Δu(x,y)Δy

MRS and Marginal Utility II

  • Marginal utility: change in utility from a marginal increase in consumption

  • Math (calculus): "marginal" means "derivative with respect to"

    • I will always derive marginal utility functions for you

MRS and Marginal Utility: Example

Example: For an example utility function

u(x,y)=x2+y3

  • Marginal utility of x: MUx=2x
  • Marginal utlity of y: MUy=3y2

MRS Equation and Marginal Utility

  • Relationship between MU and MRS:

ΔyΔx⏟MRS=−MUxMUy

MRS and Preferences

MRS and Preferences: Goods, Bads, Neutrals

  • More precise ways to classify objects:

  • A good enters utility function positively

    • ↑ good ⟹ ↑ utility
    • Willing to pay (give up other goods) to acquire more (monotonic)

MRS and Preferences: Goods, Bads, Neutrals

  • More precise ways to classify objects:

  • A good enters utility function positively

    • ↑ good ⟹ ↑ utility
    • Willing to pay (give up other goods) to acquire more (monotonic)

  • A bad enters utility function negatively

    • ↑ good ⟹ ↓ utility
    • Willing to pay (give up other goods) to get rid of

MRS and Preferences: Goods, Bads, Neutrals

  • More precise ways to classify objects:

  • A neutral does not enter utility function at all

    • ↑,↓ has no effect on utility

MRS and Preferences: Substitutes

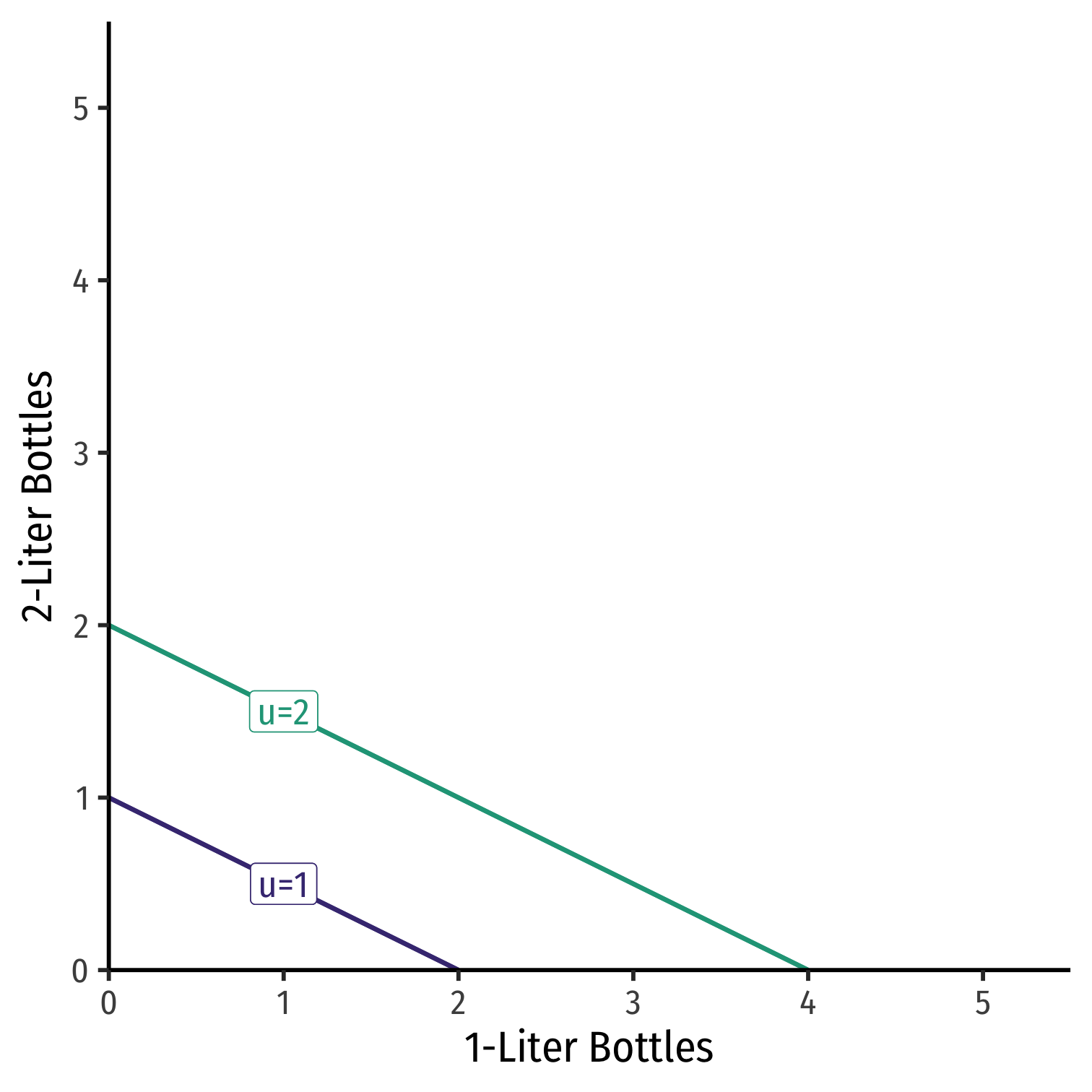
Example: Consider 1-Liter bottles of coke and 2-Liter bottles of coke

  • Always willing to substitute between Two 1-L bottles for One 2-L bottle

  • Perfect substitutes: goods that can be substituted at same fixed rate and yield same utility

  • MRS1L,2L=−0.5 (a constant!)

MRS and Preferences: Complements

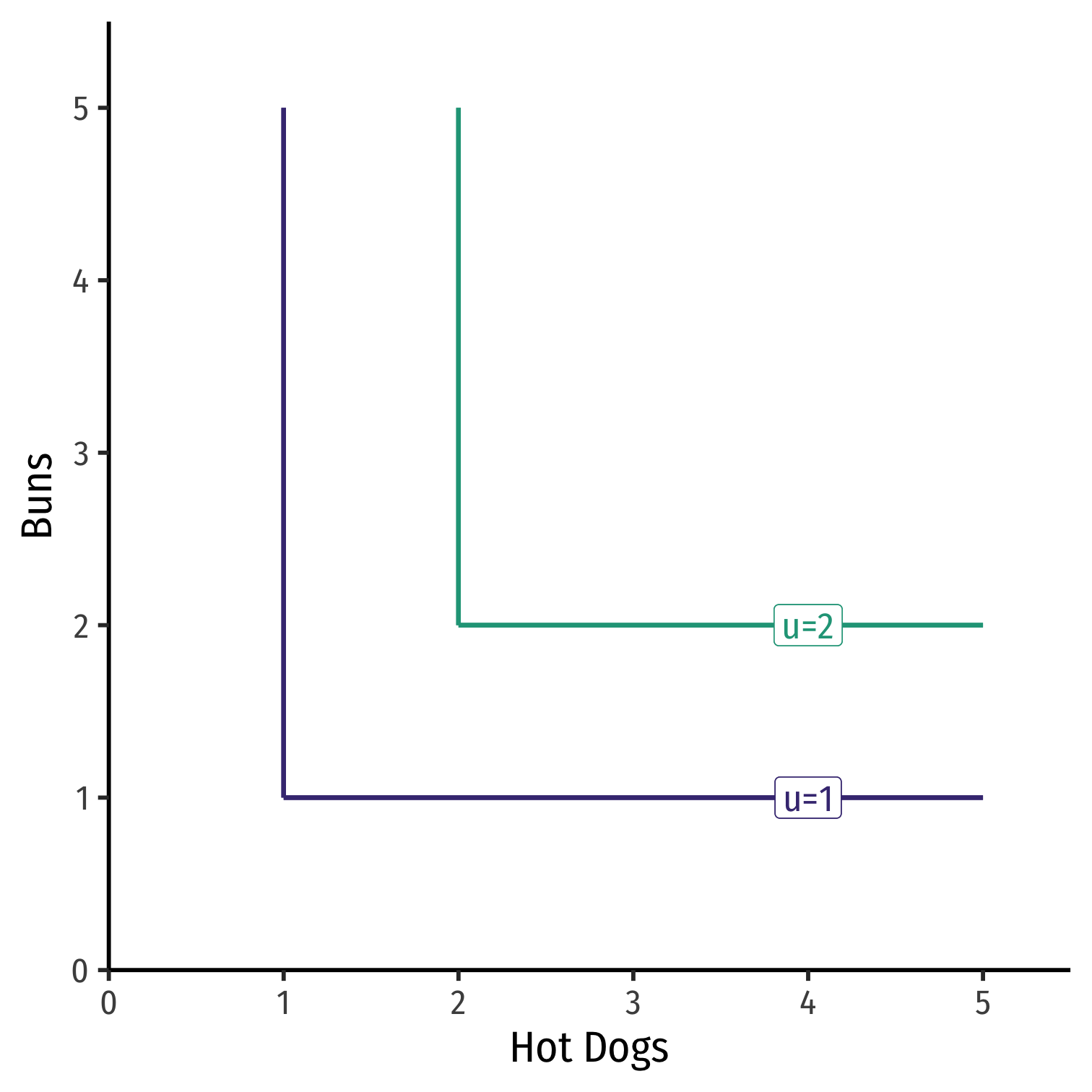
Example: Consider hot dogs and hot dog buns

  • Always consume together in fixed proportions (in this case, 1 for 1)

  • Perfect complements: goods that can be consumed together in same fixed proportion and yield same utility

  • MRSH,B= ?

Cobb-Douglas Utility Functions

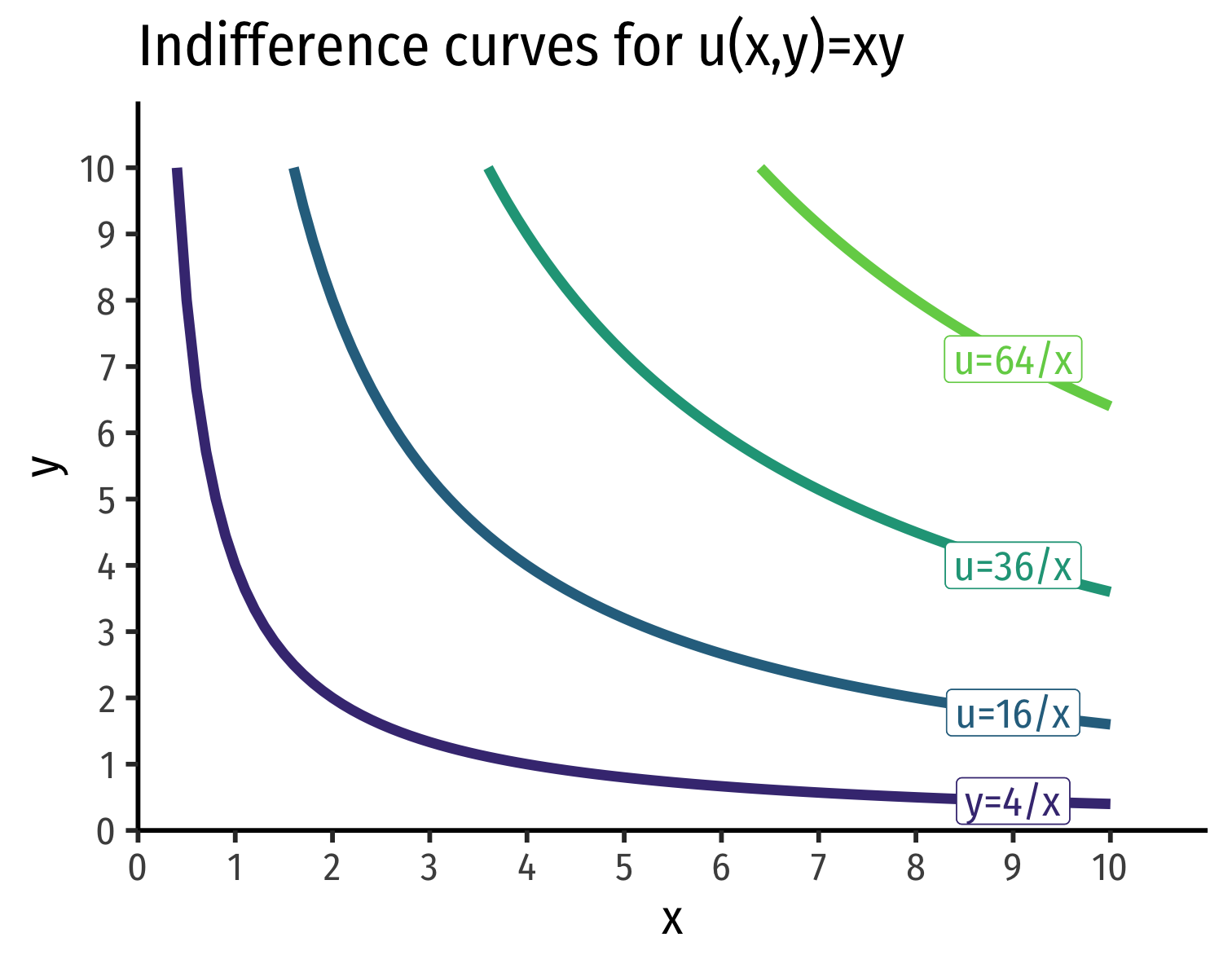
  • A very common functional form in economics is Cobb-Douglas

u(x,y)=xayb

  • Where a,b>0 (and very often a+b=1)
  • Extremely useful, you will see it often!
    • Strictly convex and monotonic indifference curves
    • Other nice properties (we'll see later)
    • See the appendix in today's class page

Practice

Example: Suppose you can consume apples (a) and broccoli (b), and earn utility according to: u(a,b)=2ab

Where your marginal utilities are:

MUa=2bMUb=2a

  1. Put a on the horizontal axis and b on the vertical axis. Write an equation for MRSa,b.

  2. Would bundles of (1,4) and (2,2) be on the same indifference curve?

Outline

Preferences

Indifference Curves

Marginal Rate of Substitution

Utility

Marginal Utility

MRS and Preferences

Paused

Help

Keyboard shortcuts

↑, ←, Pg Up, k Go to previous slide
↓, →, Pg Dn, Space, j Go to next slide
Home Go to first slide
End Go to last slide
Number + Return Go to specific slide
b / m / f Toggle blackout / mirrored / fullscreen mode
c Clone slideshow
p Toggle presenter mode
t Restart the presentation timer
?, h Toggle this help
Esc Back to slideshow